THE EASY WAY TO FACTORISE NASTY QUADRATICS

Factoring Quadratics

A Quadratic Equation in Standard Form
(a, b, and c can have any value, except that acan't be 0.)

To "Factor" (or "Factorise" in the UK) a Quadratic is to:

find what to multiply to get the Quadratic

It is called "Factoring" because we find the factors (a factor is something we multiply by)

Example:

Multiplying (x+4) and (x−1) together (called Expanding) gets x² + 3x − 4 :

So (x+4) and (x−1) are factors of x² + 3x − 4
Just to be sure, let us check:

(x+4)(x−1) = x(x−1) + 4(x−1)

 = x² − x + 4x − 4

 = x² + 3x − 4 

Yes, (x+4) and (x−1) are definitely factors of x² + 3x − 4

Did you see that Expanding and Factoring are opposites?

Common Factor

First check if there any common factors.

Example: what are the factors of 6x² − 2x = 0 ?

6 and 2 have a common factor of 2:

2(3x² − x) = 0

And x² and x have a common factor of x:

2x(3x − 1) = 0

And we have done it! The factors are 2xand 3x − 1,

We can now also find the roots (where it equals zero):

• 2x is 0 when x = 0
• 3x − 1 is zero when x = 13

And this is the graph (see how it is zero at x=0 and x=13):

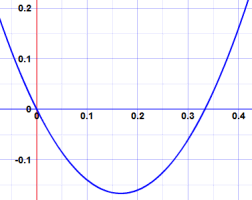
Example: what are the factors of 2x² + 7x + 3 ?

No common factors.
Let us try to guess an answer, and then check if we are right ... we might get lucky!

We could guess (2x+3)(x+1):

(2x+3)(x+1) = 2x² + 2x + 3x + 3
= 2x² + 5x + 3 (WRONG)

How about (2x+7)(x−1):

(2x+7)(x−1) = 2x² − 2x + 7x − 7
= 2x² + 5x − 7 (WRONG AGAIN)

OK, how about (2x+9)(x−1):

(2x+9)(x−1) = 2x² − 2x + 9x − 9
= 2x² + 7x − 9 (WRONG AGAIN)

Oh No! We could be guessing for a long time before we get lucky.

A Method For Simple Cases

Luckily there is a method that works in simple cases.
With the quadratic equation in this form:

Step 1: Find two numbers that multiply to give ac (in other words a times c), and add to give b.

Example: 2x² + 7x + 3
ac is 2×3 = 6 and b is 7
So we want two numbers that multiply together to make 6, and add up to 7
In fact 6 and 1 do that (6×1=6, and 6+1=7)

How do we find 6 and 1?

It helps to list the factors of ac=6, and then try adding some to get b=7.

Factors of 6 include 1, 2, 3 and 6.

Aha! 1 and 6 add to 7, and 6×1=6.

Step 2: Rewrite the middle with those numbers:

Rewrite 7x with 6x and 1x:

2x² + 6x + x + 3

Step 3: Factor the first two and last two terms separately:

The first two terms 2x² + 6x factor into 2x(x+3)
The last two terms x+3 don't actually change in this case
So we get:

2x(x+3) + (x+3)

Step 4: If we've done this correctly, our two new terms should have a clearly visible common factor.

In this case we can see that (x+3) is common to both terms, so we can go:

Start with:2x(x+3) + (x+3)

Which is:2x(x+3) + 1(x+3)

And so:(2x+1)(x+3)

Done!
Check: (2x+1)(x+3) = 2x² + 6x + x + 3 = 2x² + 7x + 3 (Yes)

Much better than guessing!

Let's see Steps 1 to 4 again, in one go:

2x2 + 7x + 3

2x2 + 6x + x + 3

2x(x+3) + (x+3)

2x(x+3) + 1(x+3)

(2x+1)(x+3)

OK, let us try another example:

Example: 6x² + 5x − 6

Step 1: ac is 6×(−6) = −36, and b is 5
List the positive factors of ac = −36: 1, 2, 3, 4, 6, 9, 12, 18, 36
One of the numbers has to be negative to make −36, so by playing with a few different numbers I find that −4 and 9 work nicely:

−4×9 = −36 and −4+9 = 5

Step 2: Rewrite 5x with −4x and 9x:

6x² − 4x + 9x − 6

Step 3: Factor first two and last two:

2x(3x − 2) + 3(3x − 2)

Step 4: Common Factor is (3x − 2):

(2x+3)(3x − 2)

Check: (2x+3)(3x − 2) = 6x² − 4x + 9x − 6 = 6x² + 5x − 6 (Yes)