Revision Notes on Quadratic Equations chapter 4 class 10 cbse

- 


       Chapter 4  (Quadratic Equations)



Quadratic Polynomial

A polynomial, whose degree is 2, is called a quadratic polynomial. It is in the form of



p(x) = ax2 + bx + c, where a ≠ 0





Quadratic Equation

When we equate the quadratic polynomial to zero then it is called a Quadratic Equation i.e. if



p(x) = 0, then it is known as Quadratic Equation.





Standard form of Quadratic Equation



Standard form of Quadratic Equation

where a, b, c are the real numbers and a≠0





Types of Quadratic Equations

1. Complete Quadratic Equation  ax2 + bx + c = 0, where a ≠ 0, b ≠ 0, c ≠ 0



2. Pure Quadratic Equation   ax2 = 0, where a ≠ 0, b = 0, c = 0





Roots of a Quadratic Equation

Let x = α where α is a real number. If α satisfies the Quadratic Equation ax2+ bx + c = 0 such that aα2 + bα + c = 0, then α is the root of the Quadratic Equation.



As quadratic polynomials have degree 2, therefore Quadratic Equations
 can have two roots. So the zeros of quadratic polynomial p(x) =ax2+bx+c is same as the roots of the Quadratic Equation ax2+ bx + c= 0.





Methods to solve the Quadratic Equations

There are three methods to solve the Quadratic Equations-





1. Factorisation Method

In this method, we factorise the equation into two linear factors and
 equate each factor to zero to find the roots of the given equation.



Step 1: Given Quadratic Equation in the form of ax2 + bx + c = 0.



Step 2: Split the middle term bx as mx + nx so that the sum of m and n is equal to b and the product of m and n is equal to ac.



Step 3: By factorization we get the two linear factors (x + p) and (x + q)





ax2 + bx + c = 0 = (x + p) (x + q) = 0

Step 4: Now we have to equate each factor to zero to find the value of x.





Now we have to equate each factor to zero to find the value of x

These values of x are the two roots of the given Quadratic Equation.





2. Completing the square method

In this method, we convert the equation in the square form (x + a)2 - b2 = 0 to find the roots.



Step1: Given Quadratic Equation in the standard form ax2 + bx + c = 0.



Step 2: Divide both sides by a





Divide both sides by a

Step 3: Transfer the constant on RHS then add square of the half of the coefficient of x i.e.the half of the coefficient of x i.e. on both sides





Transfer the constant on RHS then add square



Transfer the constant on RHS then add square

Step 4: Now write LHS as perfect square and simplify the RHS.





Now write LHS as perfect square and simplify the RHS

Step 5: Take the square root on both the sides.





Take the square root on both the sides

Step 6: Now shift all the constant terms to the RHS and we can calculate the value of x as there is no variable at the RHS.





Now shift all the constant terms to the RHS



3. Quadratic formula method

In this method, we can find the roots by using quadratic formula. The quadratic formula is





Quadratic formula method

where a, b and c are the real numbers and b2 – 4ac is called discriminant.



To find the roots of the equation, put the value of a, b and c in the quadratic formula.





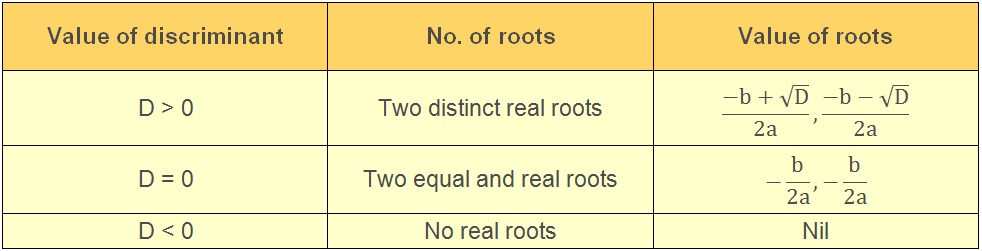
Nature of Roots

From the quadratic formula, we can see that the two roots of the Quadratic Equation are -





Nature of Roots

Where D = b2 – 4ac



The nature of the roots of the equation depends upon the value of D, so it is called the discriminant.





so it is called the discriminant



∆ = Discriminant

Value of Discriminant



                                                              by shikha kaushal
Location: Delhi, India

Popular posts from this blog

Compound Interest class 8 chapter6(notes) maths definition, formula

-
Image
Compound Interest Definition Compound interest is the interest calculated on the principal and the interest accumulated over the previous period. It is different from the simple interest where interest is not added to the principal while calculating the interest during the next period. Compound interest finds its usage in most of the transactions in the banking and finance sectors and also in other areas as well. Some of its applications are: Increase or decrease in population. The growth of bacteria. Rise or depreciation in the value of an item. Compound Interest Formula The compound interest formula is given below: Compound Interest = Amount – Principal Where the  amount  is given by: Where, A= amount P= principal R= rate of interest n= number of times interest is compounded per year It is to be noted that the above formula is the general formula for the number of times the principal is compounded in an year. If the amount is compounded annually, the amount is given as- ...
Read more

Revision notes for class 10 & 9 CIRCLES

-
Image
Revision Notes on Circle The equation of a   circle   with its center at C(x 0 , y 0 ) and radius r is: (x – x 0 ) 2  + (y – y 0 ) 2  = r 2     If x 0  = y 0  = 0 (i.e. the centre of the circle is at origin) then  equation of the circle   reduce to x 2  + y 2  = r 2 . If r = 0 then the circle represents a point or a point circle. The equation x 2  + y 2  + 2gx + 2fy + c = 0 is the general equation of a circle with centre (–g, –f) and radius √(g 2 +f 2 -c). Equation of the circle with points P(x 1 , y 1 ) and Q(x 2 , y 2 ) as extremities of a diameter is (x – x 1 ) (x – x 2 ) + (y – y 1 )(y – y 2 ) = 0.  For general circle, the equation of the chord is x 1 x + y 1 y + g(x 1  + x) + f(y 1  +y) + c = 0 For circle x 2  + y 2  = a 2 , the equation of the chord is x 1 x + y 1 y = a 2   The equation of the  chord  AB (A ≡ (R cos α, R sin α);...
Read more

Basic concept of probability

-
Image
Important Formulas - Probability or Chance Probability or Chance Probability or chance is a common term used in day-to-day life. For example, we generally say, 'it may rain today'. This statement has a certain uncertainty. Probability is quantitative measure of the chance of occurrence of a particular event. Experiment An experiment is an operation which can produce well-defined outcomes. Random Experiment If all the possible outcomes of an experiment are known but the exact output cannot be predicted in advance, that experiment is called a random experiment. Examples Tossing of a fair coin When we toss a coin, the outcome will be either Head (H) or Tail (T) Throwing an unbiased die Die is a small cube used in games. It has six faces and each of the six faces shows a different number of dots from 1 to 6. Plural of die is dice. When a die is thrown or rolled, the outcome is the number that appears on its upper face and it is a random in...
Read more